
We offer tutoring programs for students in K-12, AP classes, and college. SchoolTutoring Academyis the premier educational services company for K-12 and college students.

The two expressions have the same denominator, so we can write as one rational expression by adding the numerators together. Interested in math tutoring services? Learn more about how we are assisting thousands of students each academic year. Step 1: Add the rational expression by adding their numerators together. Both are multiplied by the LCM, so the new numerator is. The resulting rational expression can then be simplified. Then, subtract the numerators and write the difference over the LCD. Then write each expression as an equivalent expression by multiplying it by a form of 1 using the LCD. First, find the LCM of the unlike denominators to form the LCD. Subtraction with unlike denominators is similar to addition with unlike denominators, with an additional step.
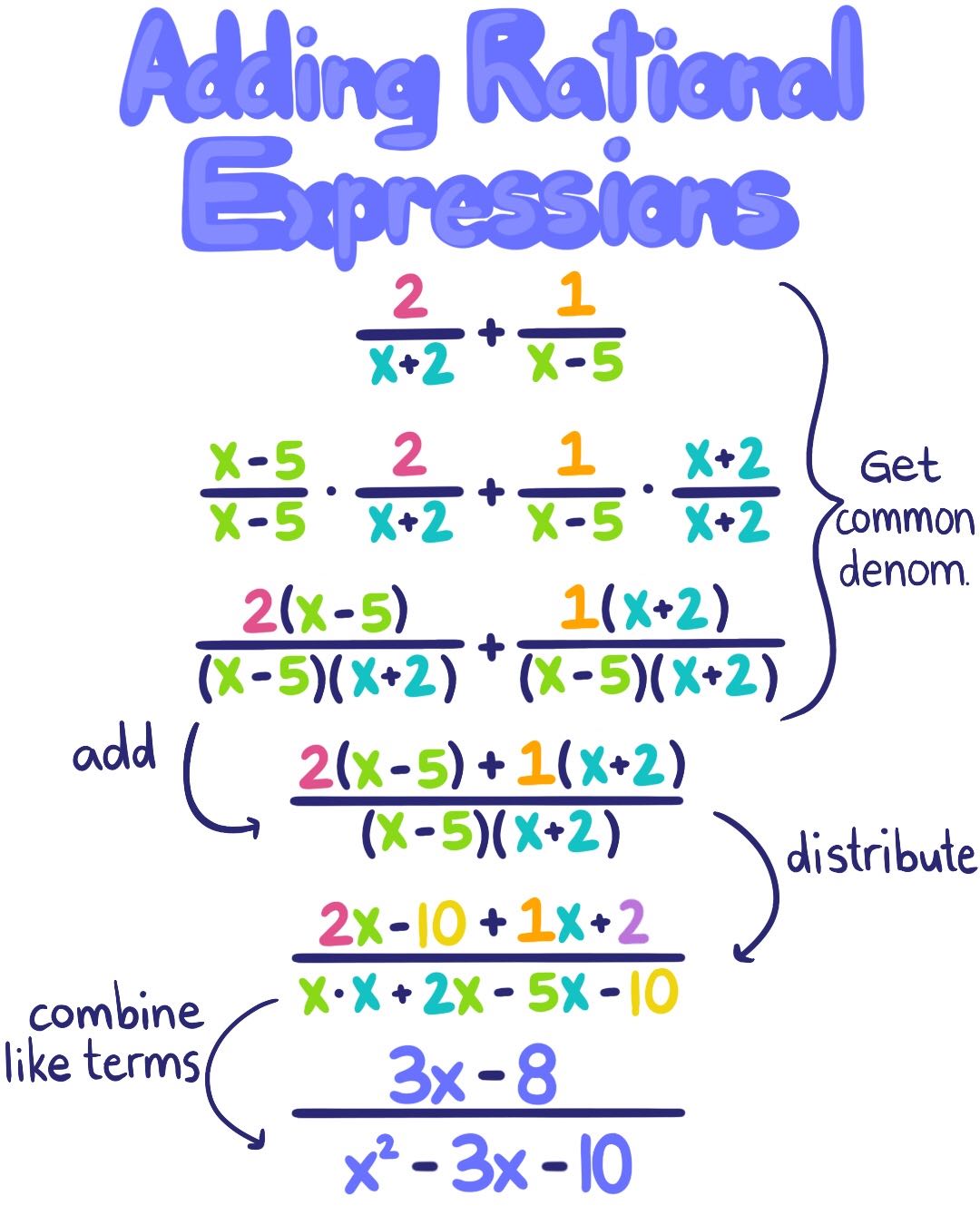
Adding expressions over like denominators, the solution is then (56x 2 +9x)/48. The number 6 factors as 2♳ and the number 16 factors as 2♲♲♲. Suppose the expressions are (7x 2)/6 and (3x)/16. Adding & subtracting rational expressions. The numerators can then be added, and the rational expression can be simplified. Lesson 8: Adding subtracting rational expressions (factored) Subtracting rational expressions: factored denominators. In order to add expressions with unlike denominators, first find the LCM of the denominators. Expressions such as (t 2+16) and (t-2) have no factors in common, so their LCM is (t 2+16) (t-2). The LCM is (y +4) (y+1) (y +1), or (y +1) 2(y +4). Adding or Subtracting Rational Expressions with Like Denominators. The first polynomial can be factored as (y +4) (y +1), and the second one can be factored as (y +1) (y +1). We seek the least common denominator, LCD, because this produces the easiest. When multiplying or dividing fractions, a common denominator is not necessary. This is what you must do when you add or subtract rational expressions. Suppose one expression is y 2 +5y +4 and the other is y 2 +2y +1. n2 + n 6 (n 2)(n + 3) n 2 (n 2) LCD (n 2)(n + 3) Find the LCD. When adding or subtracting fractions, you need a common denominator. In order to find the LCM of polynomial expressions, it is also necessary to factor them first, if they can be factored. Putting them together, the LCM of 8x 2y 2 and 12xy 3 is 24x 2y 3. Similarly, the LCM of x 2 and x is x 2 and the LCM of y 2 and y 3 is y 3. The LCM of 8 and 12 can be factored as 2♲♲♳ or 24. The coefficient 8 can be factored as 2♲♲ and the coefficient 12 can be factored as 2♲♳. Suppose the expressions are 8x 2y 2 and 12xy 3. The process is similar with expressions containing variables. Compare that to just multiplying 6♱0, or 60. Their least common multiple is 2♳♵ or 30. For example, the number 6 can be factored as 2♳, and the number 10 can be factored as 2♵. This is similar to finding the common factors of ordinary numbers without variables.
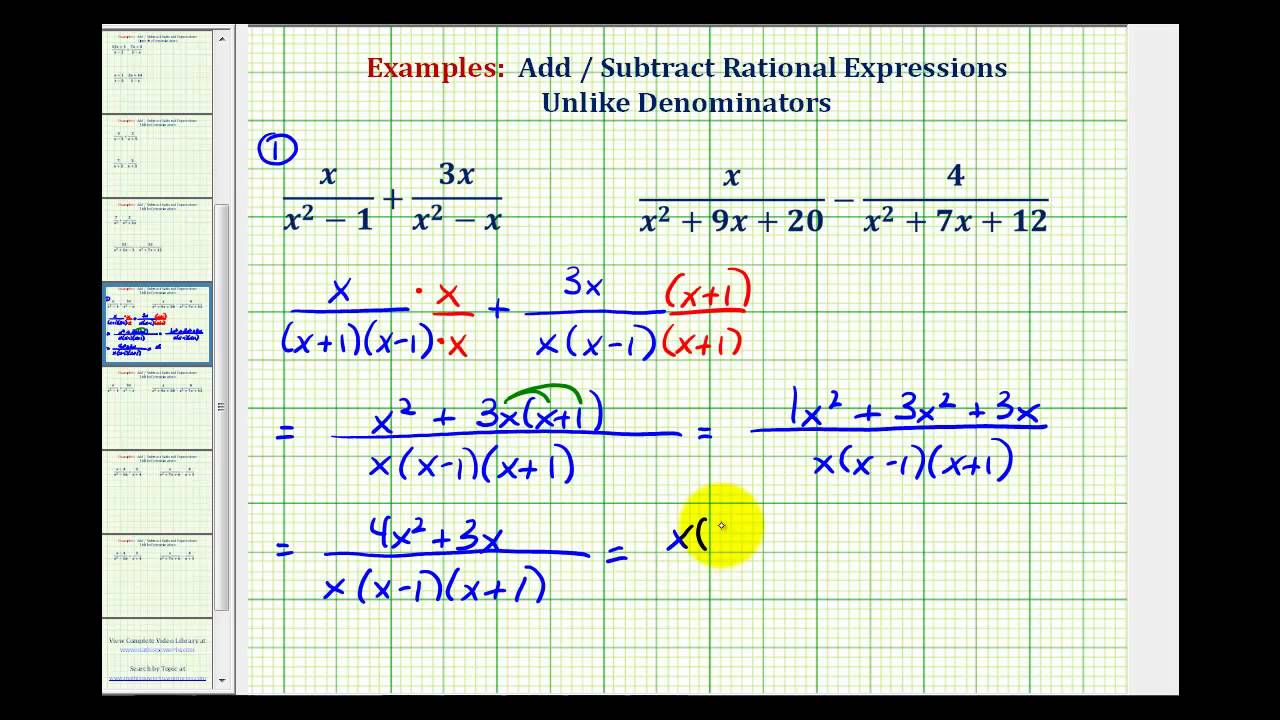
Write this sum/difference as the numerator over the common denominator. The procedure of adding or subtracting rational expressions is a lot like adding or subtracting fractions but involves rational functions or expressions. To add/subtract rational expressions with the same denominator. Adding and subtracting rational expressions is identical to adding and. To add or subtract two rational expressions with the same denominator, we simply add or subtract the numerators and write the result over the common denominator. Rational Expressions with the Same Denominator. To find the LCM of two or more algebraic expressions, first factor each expression. Objective: Add and subtract rational expressions with and without common denominators. Subtraction is the process of adding the inverse. Next, add the numerators now that the denominators are alike. Then multiply each rational expression so that it has the least common denominator. This can then form the least common denominator. The first step is to find the least common multiple (LCM) of the denominators. If the two rational expressions that you want to add or subtract have the same denominator you just add/subtract the numerators which each other.Adding rational expressions with unlike denominators is similar to adding fractions with unlike denominators.


 0 kommentar(er)
0 kommentar(er)
